This section addresses some of the main hydrogen safety concerns. Possible scenarios, including vapour cloud dispersion, ignition and hydrogen tank rupture, are discussed, along with their possible consequences. The section also identifies significant safety hazards and proposes the most important prevention measures.
Risk-based Regulatory Design for the Safe Use of Hydrogen

7. Hydrogen safety aspects
Abstract
In 2020, IFV (Instituut FysiekeVeiligheid), Netherlands, performed a review on the safety aspects associated with the release of hydrogen in confined space. The research questions in the IFV report were drawn up in collaboration with participants in the Hydrogen Community of Practice (CoP) and followed the timeline of a scenario where hydrogen is released into a confined space. Approaches to estimate the likelihood of hydrogen ignition and measures that can be taken, in order to prevent and mitigate the consequences were also provided.
In this Section, complementary to the IFV review, the main hydrogen safety aspects are discussed based on existing knowledge by theoretical, experimental and numerical research. The findings are not dedicated to a specific scenario; rather provide insight on hydrogen behaviour in case of release, dispersion and combustion. Possible safety strategies and recommendations on the safer use of hydrogen are also presented.
Hydrogen properties
Hydrogen is the simplest chemical element. It is a colourless, odourless, tasteless, flammable gaseous substance. As a fuel, hydrogen has high energy content by mass, nearly three times the energy content of gasoline—120 MJ/kg for hydrogen versus 44 MJ/kg for gasoline. However, due its low density the volumetric energy content of hydrogen is low if not compressed or in liquid state. Thus, a common practice for efficient storage, transportation and handling of hydrogen is its compression or liquefaction. This can increase the associated risks, especially, for unintended releases from pressurised storage. The increased risk, though, can be mitigated by using proper preventive and mitigation measures and performing personnel training.
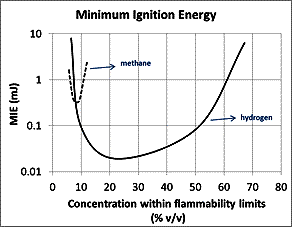
The risks of hydrogen should be compared in relation to the risks of other conventional fuels, such as natural gas (87-98% methane). Table 7.1 provides some properties of hydrogen and methane that affect their dispersion and combustion. Hydrogen has lower density, calorific value by volume and auto-ignition temperature, while it has higher heat capacity, calorific value by mass, flame temperature, laminar burning velocity and molecular diffusivity. It has wide flammability limits (4-75% v/v) and low minimum ignition energy (0.02 mJ). The MIE corresponds to concentration around 23 % v/v H2 in air. For concentration levels closer to LFL (below 10 % v/v) and to UFL (more than 55 % v/v) the ignition energy of hydrogen is higher and similar to that of methane, as shown in Figure 7.1.
Figure 7.1. Ignition energy in respect with the concentration of the fuel in air (for hydrogen and methane)
Based on the comparative table, the wider flammability limits, the lower MIE for H2 concentrations around 8-58% v/v, the lower auto-ignition temperature and the higher burning velocity and flame temperature compared to methane are the factors by which hydrogen is considered more dangerous. It should be kept in mind, though, that the associated risks of hydrogen use can be reduced to an acceptable level, comparable to other hydrocarbon fuels, by the adoption of appropriate safety measures.
Table 7.1. Comparison between hydrogen and methane properties
|
Property |
Hydrogen |
Methane |
|---|---|---|
|
Molar mass (g/mole) |
2.02 |
16.04 |
|
Vapour density (kg/m3) |
0.08 |
0.65 |
|
Specific heat capacity (kJ/kg/K) (at T='293.15' K) |
14.4 |
2.21 |
|
Lower calorific value by mass (lower heating value, weight basis) (MJ/kg) |
120 |
48 |
|
Lower calorific value by volume at 1 atm (MJ/m3) |
11 |
35 |
|
Higher calorific value by mass (MJ/kg ) |
142 |
53 |
|
Higher calorific value by volume at 1 atm (MJ/m3) |
13 |
39 |
|
Maximum flame temperature (K) |
1800 |
1495 |
|
Explosive limits (vol % in air) |
18.2-58.9 |
5.7-14 |
|
Flammability limits (vol % in air) |
4.1-74 |
5.3-15 |
|
Lower limiting oxygen concentration for combustion (vol %) |
5 |
12 |
|
Auto-ignition temperature (K) |
833.15 |
873.15 |
|
Laminar burning velocity (m/s) |
3.1 |
0.4 |
|
Molecular diffusivity in air (m2/s) |
6.110-5 |
1.610-5 |
Source: (Messaoudani et al., 2016[2]).
Vapour cloud dispersion
The accumulation and formation of flammable vapour clouds is a significant parameter in safety engineering. Hydrogen is lighter than air with a high diffusion coefficient. When hydrogen is released outdoors, wind and buoyancy force cause the mixture to rise and disperse. This behaviour is somewhat desirable for safety, as hydrogen will not accumulate near the ground, where ignition sources are more likely to be present. Similarly, in indoor/ confined spaces hydrogen tends to accumulate on the ceiling and on the top part of a facility. This rich-in-hydrogen layer below the ceiling can take on specific dimensions and thickness depending on the conditions and also flow into another part of the building. It should be noted that in compressed horizontal releases the momentum dominant region can be larger than ambient releases and buoyancy effects are evident at longer distances from the release.
Hydrogen distribution depends on the containment leak conditions, such as the leak diameter and pressure, the volume of hydrogen released and the ventilation characteristics of the location. Several experimental and numerical studies have been carried out to investigate hydrogen behaviour and understand the underlying phenomena. Next, we focus on certain studies that have provided insight on hydrogen vapour cloud dispersion, mainly in confined spaces.
(Gupta et al., 2009[3]) conducted experiments with helium release as hydrogen surrogate inside a semi-closed facility in an unventilated private garage showing that the risk (in terms of peak hydrogen concentration) is affected mostly by the total mass released rather than the release flow rate. However, variations in flow rates influence the mixing behaviour inside the garage that in turn changes the decay rates of gas concentrations. For low released volume (1.2 m3) inside a 41.3 m3 garage the peak concentration was little above the LFL (4.39 %).
The substitution of hydrogen with helium is a common practice in small dispersion experiments for safety reasons, because the two gases have similar dispersion behaviour, while helium is non-flammable.Since helium is expensive it is not appropriate for large containments. Simulation studies (He et al., 2016[4]), (Prabhakar et al., 2017[5]), (Giannissi et al., 2021[6]) compared the behaviour of the two gases and showed that the agreement between the results is very good. Thus, the use of helium as hydrogen surrogate in experiments is a valid approach and the findings are not expected to be affected. CEA performed a series of experiments in ventilated and unventilated small rooms to study helium distribution (as hydrogen surrogate for safety reasons) for several leak conditions (at ambient release pressure) (Cariteau and katschenko, 2012[7]), (Cariteau and Tkatschenko, 2013[8]). In (Cariteau and katschenko, 2012[7]) helium release through different nozzle sizes (5 and 20 mm) inside a closed facility was examined. The flow rate was ranged from 1 Nl/min to 350 Nl/min the main findings were:
Distribution depends on Riv1 number, which in turn depends on the facility volume and the leak velocity.
The higher the volume and the lower the velocity the higher the Riv.
At steady state for Riv>1 a stratified mixture is formed, for Riv<1 a stratified with homogeneous mixture on the top is formed and for Riv<<1 a homogeneous mixture is formed.
In (Cariteau and Tkatschenko, 2013[8]) helium was released through different nozzle sizes in a naturally ventilated facility. Different vent sizes were tested. The main findings are:
Distribution depends on Riv number, which in turn depends on the facility volume and the leak velocity, and on the vent size and characteristics.
Riv>1 result in a homogeneous layer on the top, for Riv<1 a homogeneous layer on the top increases, for Riv<<1 a homogeneous mixture is formed.
The highest the vertical extension of the vent more quickly formation of the homogeneous layer
Based on the above, for a facility with no ventilation, the larger the leak velocity and the smaller the volume of the facility, the more homogeneous distribution inside the facility. For a ventilated facility with one vent, the larger the leak velocity and the smaller the volume of the facility the larger the homogenous layer in the upper part of the facility. The greater the vertical extension of the vent size the slower the formation of the homogeneous layer. Moreover, the thickness of the homogenous layer decreases with decrease of vertical extension of the vent and a more stratified mixture is formed in the lower part of the facility. In terms of safety, for the same total mass of hydrogen inside the enclosure a stratified mixture can lead to more severe explosions compared to homogeneous mixtures (Skjold et al., 2019[9]).
The pressure at the point of the release is another factor that influences hydrogen distribution. High-pressure releases will result in under-expanded jets and sonic flows. (Hooker, Hoyes and and Hall, 2014[10]) conducted experiments with compressed hydrogen release inside a ventilated facility and concluded that sonic flows lead to relatively uniform hydrogen distributions compared with subsonic releases at similar flow rates. The aim of the experiments was to evaluate the ventilation effect on hydrogen dispersion and thus different passive vent configurations were tested. The facility was also exposed to naturally varying wind and mimicked a warehouse with forklift trucks, a room with fuel cells, or a hydrogen storage room. The main findings of this work relevant to ventilation efficiency can be found in the section covering Ventilation.
High turbulence in the area of release can also affect the dispersion and lead to homogeneous mixtures (IFV, 2020[11]). Thus, the presence of obstacles in the environment where the leak takes place influences the dispersion of hydrogen but to a lesser degree compared to leak conditions. Obstacles in laminar flow can generate turbulence resulting in better mixing and uniform mixtures below the ceiling. On the other hand, in turbulent flow, obstacles disrupt vortices, causing the hydrogen jet to lose energy and decelerate, therefore reducing uniformity in distribution and leading to higher concentrations beneath the ceiling increasing the risks of explosion.
To define the hazardous zones, the downwind distance that mixture with a concentration above the Lower Flammability Limit (LFL) extends is a significant parameter. Based on recent experiments performed by Pro-Science (Grune et al., 2021[12]), for release pressure around 11.5 bar (ṁ=5 g/s, d=4 mm) the LFL distance of free jet is 2.7 m. For release pressure around 115 bar (ṁ=5 g/s, d= 1 mm) the LFL distance of free jet is little less than 2.5 m, while for the 21bar pressure (ṁ=1 g/s, d= 1 mm) the LFL distance is around 1.1 m. The results verify the dependence of the LFL distance to both the operating pressure and the leak size. Along with the experiments in a stagnant environment, (Grune et al., 2021[12]) conducted experiments in presence of co-flow, counter-flow and cross-flow highlighting the reduction of LFL distance with all three ventilation configurations (see Ventilation section).
Simple correlations can be used for a first estimation of the LFL distance. Based on the similarity law (Chen and Rodi, 1980[13]) in unobstructed jet releases in a stagnant environment the concentration at the jet centerline depends on the nozzle diameter and density. The following relation gives the released gas mass fraction with respect to the distance from the nozzle:
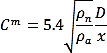
where Cm is the hydrogen mass fraction, ρ is the density, D is the nozzle diameter and x is the axial distance. Index n is for nozzle conditions and α for air. The above equation is valid provided that buoyancy effects are negligible. Thus, for buoyant gas releases, like hydrogen release, it stands only for distances close to the nozzle where momentum is dominant. Based on the similarity law, LFL distance increases proportional to leak diameter, so by decreasing the leak size you decrease proportionally the safety distance in unobstructed releases. Other factors like ventilation and wind can also affect the LFL distance and should be considered.
The similarity law can be expanded to compressed releases, if the density at the nozzle is properly computed. Considering that in high-pressure releases the pressure at the nozzle is higher than ambient, and the density of a gas increases with pressure, the similarity law implies that the axial distance of the LFL concentration is increased with increasing release pressure. This in turn means longer and more hazardous flames. Calculations in elab (https://fch2edu.eu/home/e-laboratory/)2 show that for hydrogen release through a 5 mm nozzle from 350 bar the 4% (LFL) distance is 32.6 m, while for ambient release the LFL extends only to 2.5 m.
Integral models can be also employed to predict the gas dispersion in simple geometries, like in HyRAM software3 (Ehrhart et al., 2021[14]), (Groth and and Hecht, 2017[15]). More sophisticated tools, like CFD codes, can be used to predict hydrogen dispersion and flammable distances in the 3D domain in complex geometries, e.g. in presence of obstacles, where simple correlations and models will not work properly.
In unignited releases of hydrogen, the Pressure Peaking Phenomenon (PPP), first introduced by (Brennan and Molkov, 2013[16]) is also of concern in closed facilities, as it can result in overpressure exceeding enclosure or building structural strength limit in case of sufficiently high hydrogen release rate. Recent experiments by USN (Lach et al., 2020) demonstrated the relationship between ventilation area, enclosure volume, and release rate which may result in significant overpressures in an enclosure (max. measured overpressure of the examined cases was 8.05 kPA). The maximum release rate, hence TPRD diameter, should be regulated in RCS to protect humans and surroundings. The mitigation of the PPP can be achieved by either decreasing the TPRD diameter or increasing the area of the vents. However, the PPP mitigation measures should also account for tank-TPRD system rupture in case of fire. Similar work of (Lach and Gaathaug, 2021[17]) with ignited hydrogen release in confined space demonstrated that PPP from the jet fire is more hazardous compared to the PPP for unignited release from the same source, as it results in higher overpressures.
Ignition
In case of hydrogen leak, a flammable cloud may be formed that could be ignited. It has to be noted that 28.65 g of trinitrotoluene (TNT) is energetically equivalent to 1 g of hydrogen. Moreover, hydrogen is more susceptible to deflagration-to-detonation transition (DDT) than hydrocarbons (Rigas and P., 2013[18]) and (Lins and de Almeida, 2012[19]). The properties that play decisive roles in ignition are: flash point temperature, auto-ignition temperature, minimum ignition energy (MIE), the lower flammable limit (LFL) and the upper flammable limit (UFL). For ignition to occur certain criteria must be met: the gas or vapour cloud should be within its flammability range and an ignition source of sufficient energy should be present.
Ignition can be categorised as immediate and delayed ignition depending on the time when ignition occurs. Immediate ignition happens when a released jet ignites immediately after its leakage and results in jet fires or a fireball. The jet flame may be invisible and can injure people, damage objects and buildings, and possibly create secondary effects.
Delayed ignition is considered when the ignition occurs at some time after the leakage starts and when the vapour cloud has already been diffused and mixed with surrounding air. It is caused by an ignition source remote from the point of release. Delayed ignition can result in flash fire, deflagration and/or detonation. If the vapour cloud ignited is in the open, it could create a flash fire and in most cases no overpressure effects are to be expected- only heat effects (IFV, 2020[11]). In a confined space, an overpressure may be created, which could cause major damage in humans and buildings.
Ignition sources
For ignition to occur in the presence of a hydrogen flammable cloud, an ignition source has to be present to initiate the explosion. Some potential ignition sources are:
Mechanical and electrical sparks
hot surfaces, flames
frictional heating
adiabatic compression and shock waves
electrical equipment, especially non-flameproof motors
static electricity
radio waves
The most common cause of ignition is an electrostatic discharge. Compared to spark discharges, brush discharges from electrical motors (having energy < 4mJ) are much less likely to cause an explosion (IFV, 2020[11]).
However, an early ignition can prevent elongated cloud formation that could lead to detonation. Although full elimination of ignition sources is a safe approach, it is not always possible and it should be considered in conjunction with the fact that if the cloud is not immediately ignited very large flammable clouds may be formed, especially in non-ventilated closed spaces, with the potential of severe explosions. At this point it should be noted that early controlled ignition (flaring) is usually not recommended as a safety measure in household applications of hydrogen. Early venting and hydrogen escape outdoors at high elevations without deliberate ignition should be sufficient and is the recommended approach rather than flaring. However, for depressurisation of pipelines flaring can be considered as the preferred option.
Ignition probability
The estimation of ignition probability is a key step in the quantitative risk analysis. To define the ignition probability for hydrogen releases we need to define the probability of a flammable atmosphere existing and then the probability of ignition of the flammable atmosphere.
In the study by (Moosemiller, 2011[20]), the probability of immediate ignition is the sum of the probability of auto-ignition and the probability of static discharge. Given that release temperature would be practically always lower than the auto-ignition temperature of hydrogen the probability of auto-ignition is assumed zero (IFV, 2020[11]). The ignition of static discharge depends on minimum ignition energy and release conditions, such as process pressure or release rate. Thus, the ignition probability for hydrogen release is given by,
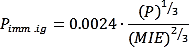
where P is the pressure in psi and MIE is in mJ.
A delayed ignition probability is significantly affected by the vapour cloud size, the release duration and the vapour cloud location and the number of available ignition sources (Zhu, Jiang and Yuan, 2012[21]). In the study by (Moosemiller, 2011[20])a relationship has been developed in which the approximate probability of delayed ignition can be determined (IFV, 2020[11]) by,
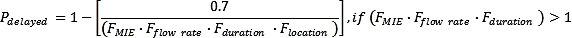
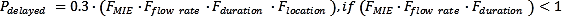
Flocation in the above equations consists of the several sub-factors, such as Froom volume, Fventilation, Fstrategy and Felectr. Classification. Relations to calculate these factors can be found in (IFV, 2020[11]).
In Purple book (Guidelines for Quantitative Risk Assessment in the Netherlands, Haag and Ale, 2005) the probability of delayed ignition caused by an ignition source accounts for the probability of the ignition source to be present and, thus, expressed by the following equation,
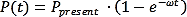
where P(t) the probability of an ignition in the time interval 0 to t (-), Ppresent the probability that the source is present when the cloud passes (-), ω the ignition effectiveness (s-1), t time (s).
The ignition effectiveness, ω, can be calculated given the probability of ignition for a certain time interval. The Purple book gives a table with the probability of ignition for a time interval of one minute for a number of sources. However, those numbers are not well established and should only be used as a guideline.
Finally, in Purple book formulae to calculate ignition probability for a road or railway near the establishment or transport route under consideration and the probability of an ignition for a grid cell in a residential area in the time interval 0 to t are also given. For the ignition probability for a road or railway the average traffic density should be determined. The average traffic density is calculated based on the number of vehicles per hour, the length of the road or the railway section and the average velocity of the vehicle. For the ignition probability for a grid cell in a residential area the average number of people present in the grid cell is used. For more details, please refer to the Purple book.
Even though it is difficult to calculate the total as well as partial probabilities, because of lack of sufficient data (Tchouvelev et al., 2006[22]) and HYSAFE 2007 (Rodsaetre and Holmefjord, 2007[23]) have proposed a hydrogen ignition probability based on the leak rate and the pressure. Gas accumulation by confinement or extensive congestion is not considered. The proposed probabilities are presented in Table 7.2. However, in 2015 The UK Health and Safety Laboratory (HSE) concluded that no direct ignition probabilities could be derived for hydrogen from available literature.
Table 7.2. Hydrogen ignition probabilities
|
Hydrogen leak rate (kg/sec) |
Immediate ignition probability |
Delayed ignition probability |
Hydrogen leak rate (kg/sec) |
Immediate ignition probability |
|---|---|---|---|---|
|
<0.125 |
0.008 |
0.004 |
0.01-0.1 |
0.001 |
|
0.125-6.25 |
0.053 |
0.027 |
0.01-1 |
0.001 + 0.001when P>100 bar |
|
>6.25 |
0.23 |
0.12 |
1-10 |
0.01 + 0.01 when P>100 bar |
|
Average |
0.097 |
0.05 |
>10 |
0.1 + 0.01 or 0.02 |
Note: These figures are generic. In detail, the probabilities are application and location specific.
The total ignition probability is the sum of the average probabilities for immediate and delayed ignition. Based on (Tchouvelev et al., 2006[22])proposed probabilities of Table 7.2 the total ignition probability is estimated to be 14.7%. This provides a complementary 85.3% probability of not igniting in case of accidental leak.
The probabilities proposed by (Tchouvelev et al., 2006[22]) are also used in (LaFleur et al., 2017[24]) and implemented in HyRAM risk tool.4
Consequences
In the case of ignition of a hydrogen cloud depending on the ignition delay the consequences could be jet fire, flash fire deflagration or detonation (see Ignition section). Ignition location also influences the explosion, as different conditions are met at different distances from the release (Houf et al., 2012[25]). Hydrogen concentrations determine the direction and spread of hydrogen flames. Flames burn upwards at 4 % v/v, since the propagation velocity of the flame is lower than the speed at which the hydrogen-air mixture rises, sideways at 6 % v/v and in all directions at 9 % v/v and over. The available amount of space necessary to develop the flame front is determined by the hydrogen concentration and the location of the ignition source in the confined space. The speed of hydrogen molecules in the plume travel is important. If the speed is greater than the speed at which hydrogen burns, the flame will not return in the direction of the release point. Therefore, upon ignition at LFL, only a fraction of hydrogen present burns, very little heat is produced and there is no build-up of pressure (Molkov, 2012[26]).
The explosion of a hydrogen-air mixture cloud results in the formation of a pressure wave. Hydrogen concentrations of up to 10 vol % result in hardly any overpressure. A mixture with near-stoichiometric conditions (30% v/v) is among the worst-case scenarios keeping all other parameters the same. Generally, concentration levels ranging from 25-42 % v/v are considered of great risk as high burning velocities are developed resulting in high overpressures. The magnitude of the generated overpressure in a confined space depends inter alia on hydrogen concentration, size and geometry of the space, the presence and size of any openings and the level of congestion of the environment.
In the deflagration of a free hydrogen-air gas cloud, the maximum overpressure is in the order of 10 kPa. An overpressure of 7 kPa is still considered not dangerous, as it is the threshold of people falling down to the ground (HySafe, http://www.hysafe.org, Hydrogen fundamentals).
To assess the magnitude of consequences in case of hydrogen ignition, i.e. pressure and thermal hazards, several experimental and modelling studies have been performed in the past. Based on a non-exhaustive literature review on hydrogen combustion and fire experiments, next, we discuss some of these studies and their main findings in terms of safety. More discussion and dedicated conclusions to the specific scenarios/applications are given in Mapping Exercise section.
Pressure hazards
In 2019, GEXCON performed gaseous hydrogen release inside a container with obstacles and vented explosions (Skjold et al., 2019[27]), (Skjold et al., 2019[28]). In (Skjold et al., 2019[27]) a homogenous mixture of 15 % v/v H2 was ignited inside a 20 m3 ISO container with openings. The effect of obstacles was also examined. The lean mixtures and simple geometry configuration resulted in weak explosions and modest effect of the obstacle on flame acceleration and pressure development. The maximum measured overpressure was 7.7 kPa with pressure impulse 530–620 kPa∙ms and was developed in the presence of obstacles. In (Skjold et al., 2019[28]) the vented explosion of non-homogeneous mixture was examined and the main findings of the work in terms of safety are:
A stratified mixture is produced, which is more evident after the end of the leak, with peak values lower than near-stoichiometric mixture (about 15-28 % v/v in the deployed sensors).
The stratified mixture inside confined spaces can lead to more severe explosions.
Obstacles, like the pipe rack that can be used as hydrogen storage, can somewhat increase overpressure.
Maximum overpressure 60 kPa (when the mixture ignited at peak concentration). The overpressure at most sensors is 40 kPa, which means 50% probability of eardrum rupture.
For H2 release experiments from storage at a pressure 4 MPa through a 12 mm diameter hole and storage volume 5 m3 (Daubech et al., 2015[29]) the LFL jet centreline distance was more than 17 m. Ignition of the cloud at 1.8 m, where hydrogen concentration was around 30% (near-stoichiometric mixture), generated a maximum overpressure of 8 kPa at 2 m downstream the ignition position (threshold of skin lacerations by missiles, window glass shatters, light injuries from fragments).
Experiments performed by FM Global (Bauwens, Chaffee and Dorofeev, 2011[30]) inside a 64 m3 chamber to compare the combustion consequences among hydrogen, methane and propane. The experiments involved ignition of 18% vol. hydrogen–air, 9.5% methane–air and 4.0% propane–air mixtures at two different locations and two vent sizes. These experiments showed that, despite having similar laminar flame speeds, the hydrogen mixtures propagated at flame speeds significantly higher than the methane and propane mixtures. Due to the higher propagation speeds of the hydrogen flames, significantly higher overpressures (up to 30kPa) were generated during the vented explosions when compared to the methane and propane mixtures. Moreover, the larger the vent size the lower the pressure was.
The above finding on the dependence of overpressure on vent area is also supported by (Chen et al., 2020[31]), who showed that the deflagration overpressure decreases with the increase in the vent area. The raised vent location may affect the pressure development during explosion venting. Moreover, in the same study it was shown that with the increase of the volumetric blockage ratio inside the chamber, the maximum overpressure and flame velocity become higher. The behaviour of the non-homogenous hydrogen deflagration is controlled by the maximum hydrogen concentration in the chamber, but the maximum overpressure and flame velocity for the non-homogenous mixture are slightly lower than those for the homogenous mixture with the same maximum concentration. The maximum overpressure measured inside the empty chamber increases as hydrogen concentration increases from 15.3% to 20.2%.
The effect of blockage ratio on overpressure was also examined and verified by (Schiavetti and Carcassi, 2021[32]) examining different obstacle configurations. It was shown that increasing the blockage ratio the maximum overpressure also increases, nevertheless, for the tested configurations, the effect of repeated obstacles on the increase of maximum peak pressure is higher than that of increasing blockage ratio.
To similar conclusions was led the study of (Chen et al., 2020[33]) based on experiments inside a 27 m3 chamber with different vent areas and different mixtures (uniform hydrogen-air mixtures with concentrations ranging from 15 % to 21 % and stratified mixtures with maximum concentrations equal to average values of uniform mixtures). Among their findings were those: both maximum hydrogen concentration and hydrogen inventory inside the chamber affect the external pressures during non-homogenous hydrogen deflagrations, but the maximum hydrogen concentration is the dominant factor. Higher volumetric blockage ratio leads to higher external pressures. The obstruction has a significant influence on the external pressures during non-homogenous hydrogen deflagrations when the volumetric blockage ratio inside the chamber is large enough, but its effect is much stronger for the homogenous deflagration.
Fire and thermal hazards
The above studies examined the pressure hazards in case of hydrogen ignition. However, another consequence is fire and thermal hazards. The radiation heat flux in the case of a hydrogen fire may harm people and buildings depending on its magnitude and its dose.
In the case of an expanded jet fire the hydrogen flame length is proportional only to the leak diameter based on (Hottel and and Hawthorne, 1949[34]) and (Hawthorne, Weddell and and Hottel, 1949[35]). However, the work of (Kalghatgi, 1984[36]) that studied experimentally both with subsonic and sonic hydrogen jet flames concluded that for both flows: 1) the flame length increases with the mass flow rate at a fixed diameter, and 2) the flame length increases with the diameter at fixed mass flow rate.
In e-lab (https://fch2edu.eu/home/e-laboratory/) there is a validated correlation available that calculates the flame length and hazard distances depending on chosen hazard criteria for both expanded and under-expanded jets (subsonic and sonic flows, respectively). For instance, for storage at very high pressure, 350 bar, ambient temperature and 2 mm nozzle the flame length is 5.2 m, the no harm separation distance (70oC) is 18.2 m and the pain limit separation distance (5 mins, 115oC) is 15.6 m. The HyRAM tool also has the capability to calculate thermal hazards (heat flux and temperature maps) from hydrogen jet flames using well validated models of flame physics.
Another concern of hydrogen is the risk of transition to detonation (detonation propagation velocities are higher than speed of sound), which will under certain circumstances create a significant pressure impulse to the human and surrounding civil structures. A H2-air mixture deflagration (subsonic burning velocities) is likely to eventually develop into detonation during accidents because hydrogen has shorter Deflagration to Detonation Transition (DDT) distance compared to other fuels (Li, 2018[37]), (Li et al., 2021[38]). Thus, in case of delayed ignition, where better mixing is achieved, or if the combustion is enhanced by external obstacles, the deflagration could develop into detonation with severe consequences.
Studies for hydrogen detonation in confined spaces have been performed with the aim to estimate the threshold for onset of detonation and the generated overpressure (Groethe et al., 2007[39]), (Kuznetsov et al., 2015[40]). However, no generic results are able to be extracted as they vary with the experimental conditions. For instance, in the experiments of (Groethe et al., 2007[39]) involving hydrogen combustion in a sub-scaled tunnel with the hydrogen premixed with air and confined in a plastic film barrier the maximum overpressure was 150 kPa and was measured at the outlet of the tunnel. On the other hand, in (Kuznetsov et al., 2015[40]) an experiment was conducted in a flat semi-confined layer with gradient hydrogen concentration and the measured overpressure was in the order of magnitude of 1 MPa.
Tank rupture
In the event of fire and failure of TPRD activation or unaffected TPRD due to localised fire, hydrogen tanks can rupture causing harm to humans and damages to structures. The level of harm and damages is assessed by pressure effects of the blast wave (pressure and impulse) and thermal effects by the fireball. Tank rupture is caused by pressure increase inside the tank that could not successfully be vented. Fire close or around the tank can lead to tank rupture.
(Makarov et al., 2021[41]) presented a brief review on hydrogen tank rupture experiments and the resulting fireball size. Two fire tests were performed in the USA (Weyandt, 2005[42]), (Zalosh and Weyandt, 2005[43]), (Weyandt, 2006[44]), (Zalosh, 23–27 April 2007[45]). The tanks considered in these tests were Type III5 and Type IV6 with volume 72.4 and 88 lt and pressure 34.3 MPa and 31.8 MPa, respectively. Two more experiments were performed in Japan (Yosuke et al., 2006[46]) on tanks Type III and IV with nominal working pressure 70 MPa. Finally, two tests with compressed hydrogen Type III tanks rupture in a fire were conducted by (Shen et al., 2018[47]). At both tests the tank pressure was 35 MPa.
The maximum fireball diameter based on the above experiments was 24 m resulting from 35 MPa storage pressure and 88 lt volume (1.89 kg). The time that tank withstood rupture varied based on experimental conditions from 6 min and 27 sec to 21 min and 21 sec.
(Makarov et al., 2021[41]) also presented correlations for the calculation of fireball size based on hydrogen tank mass showing a positive correlation. A conservative model that correlates fireball size from high-pressure gaseous hydrogen tank ruptures with hydrogen mass in power ⅓ is suggested in (Makarov et al., 2021[41]).
The leak-no-burst (LNB) safety technology (Molkov, Makarov and Kashkarov, 2019[48]) for explosion free in a fire tank that has been developed by HySAFER Centre of Ulster University may resolve the issue with TPRD failure, as it does not require TPRD (TPRD-less tank). This, in turn, reduces drastically the risk of blast wave, fireball, long flame and pressure peaking phenomenon in confined spaces. This technology provides a level of risk of hydrogen-powered vehicles below that of the fossil fuel vehicles (HyResponder, 2021[49]). The main breakthrough of the LNB technology is that in case of fire hydrogen leaks through tank walls safely as insignificant leak before tank walls exceed the load bearing limit preventing rupture from happening. This is achieved by using at least two composites with different thermal properties, an external part with lower thermal conductivity and an internal part with higher thermal conductivity. The thermal parameters of the liners and their thickness are such to allow melting of the liner before resin decomposition front reaches the load-bearing fraction of the wall thickness. As an additional safety measure, TPRD can also be integrated, but with a much smaller diameter resulting in the creation of a smaller flammable cloud in case of activation. The prototype of this technology has been successfully tested.
Safety strategies
The design and implementation of efficient safety strategies in hydrogen applications and infrastructures is a key element for the safer use of hydrogen technology. There are several prevention and mitigation measures that can be applied for hydrogen following certain criteria to address its behaviour. The principle of safe-by-design, e.g. for HFCV, is the fundamental risk prevention approach. The safe-by-design aims at addressing safety issues during the R&D and design phases of new technologies and can potentially reduce the need of mitigation measures, maintenance, etc. Nonetheless, since it is not always possible to exclude the possibility of equipment failure, human error or malicious attacks, the design of measures to reduce the consequences in case of an accident is mandatory.
General recommendations on safety strategies for hydrogen technology are:
reduction of the inventory in storage facilities on-site refuelling stations
use of safety distances
installation of safety valves, such as excess flow valve, emergency control valve, pressure indicators in compressors
reduction of the TPRD size (while using proper fire resistance to tank) to avoid hydrogen concentrations above LFL on the ceiling
oblique TPRD orientation, e.g. at 45 degrees downwards
perform regular inspections and maintenance in all components (and especially joint parts) in hydrogen systems to reduce the risk of a leak
proper design, installation, use and maintenance of detection sensor and alarm system
ventilation (natural or mechanical)
ensure sufficient distance of main tunnel and elements, like dust collectors and exhaust fans, that can trap hydrogen in flammable concentrations
elimination of ignition sources, if possible, close to the ceiling, where accumulation of hydrogen is expected
limit congestion inside closed facilities as high blockage ratio accelerates the flame and leads to higher deflagration overpressure
avoid obstacles on the ceiling that could lead to accumulation of hydrogen above LFL
proper installation of fire protection walls and barrier walls in HRS
use of fire suppression systems, such as water mist to mitigate the consequences in case of fire and explosion and/or prevent the fire spreading
The next sections present further details on the installation of detection sensors and on ventilation based on existing knowledge.
Detection sensors
Hydrogen detection sensors are risk mitigation devices. They can detect a flammable atmosphere in case of an accidental leak and dictate/initiate certain actions, like activation of shutdown valves or activation of the alarm system. Optimal installation of the sensors includes the selection of the appropriate sensor technology type, number and positioning, and their calibration and maintenance. General recommendations on the installation of hydrogen detection sensors are:
Be able to detect hydrogen leakage and isolate the supply in an enclosed space before the hydrogen concentration reaches 15% (when more severe consequences occur if ignited). Generally, low alarm levels need to be considered equal to 10% of the LFL and/or 25% of the LFL.
Sensors should not be placed on a direct path of the airflow from the air inlet to the exhaust fan.
Locations close to the floor may not be practical since their expected concentration levels are on the borderline of practicality and reliable sensor detection threshold.
Locations below the enclosure ceiling thus not obstructed by the ceiling piping and lighting fixtures or other objects are preferred.
Use simultaneously different detection technologies, such as ultrasonic, electrochemical, etc. (HSE, 2017[50]) in compliance with ATEX regulations. Application of different gas/leak detection algorithms in addition to the use of a basic alarm threshold (e.g. low and high) is also recommended by UK HSE (HSE, 2017[50]).
Ventilation
Ventilation is considered a good practice to control health and safety hazards. Ventilation can be either natural or mechanical (using systems which are in compliance with ATEX regulations). Proper ventilation design and operating conditions are the key factors for an efficient system and are determined by the characteristics of hydrogen dispersion and combustion.
The EU-funded project, HyIndoor (www.hyindoor.eu), whose aim was to develop safety design guidelines, engineering tools and RCS recommendations for the safer use of hydrogen indoors, presented a series of ventilation strategies based on the conducted research. Next, we present some recommendations based on HyIndoor and other existing knowledge (Grune et al., 2021[12]), (Giannissi et al., 2015[51]), (Giannissi, Tolia and Venetsanos, 2016[52]):
Large openings enhance ventilation (and the overpressure in case of ignition decreases with increasing vent size)
Vents with large vertical extension size are preferred:
The larger the leak velocity and the smaller the volume of the facility the larger the homogenous layer in the upper part of the facility with one vent.
The larger the vertical extension of the vent size the slower the formation of the homogeneous layer.
Thus, vents with large vertical extension size promote the formation of homogeneous layers and provide better ventilation compared to vents of the same area but horizontally stretched (height < length).
Multi-vent configurations provide much more efficient ventilation than single-vent configurations
The larger height difference between multiple vents is, the more efficient is passive/natural ventilation
Vertically stretched vents (height > width) provide better ventilation compared to horizontally stretched vents of the same area based on CFD simulations
For single vent configuration, a wall vent can provide more efficient ventilation than a chimney vent of equal area
If practical, the ventilation system should be designed to prevent concentrations exceeding LFL for realistic expected hydrogen release rates
Co-flow, counter-flow and cross-flow can reduce the distance that mixture within H2 lower flammability limit (LFL) extends
External wind can either enhance or hinder ventilation depending on the vent configuration and the wind direction in respect to the vent. Several vents distributed on all sides of the enclosure (both at the top and the bottom) help to ensure that wind would enhance ventilation regardless of its direction
Simple models can give reasonable predictions in multi-vent configurations.
References
[30] Bauwens, C., J. Chaffee and S. Dorofeev (2011), “Vented explosion overpressures from combustion of hydrogen and hydrocarbon mixtures”, International Journal of Hydrogen Energy, Vol. 36/3, pp. 2329-2336, https://doi.org/10.1016/J.IJHYDENE.2010.04.005.
[1] Bjerketvedt, D., J. Bakke and K. Van Wingerden (1997), “Gas explosion handbook”, Journal of Hazardous Materials, Vol. 52/1, pp. 1-150, https://doi.org/10.1016/S0304-3894(97)81620-2.
[16] Brennan, S. and V. Molkov (2013), “Safety assessment of unignited hydrogen discharge from onboard storage in garages with low levels of natural ventilation”, International Journal of Hydrogen Energy, Vol. 38, pp. 8159-8166.
[7] Cariteau, B. and I. katschenko (2012), “Experimental Study of the Concentration Build-up Regimes in an Enclosure without Ventilation International Journal of Hydrogen Energy”, International Journal of Hydrogen Energy, Vol. 37/22, pp. 17400–408.
[8] Cariteau, B. and I. Tkatschenko (2013), “Experimental Study of the Effects of Vent Geometry on the Dispersion of a Buoyant Gas in a Small Enclosure”, International Journal of Hydrogen Energy, Vol. 38/19, pp. 8030–38.
[13] Chen, C. and W. Rodi (1980), Vertical Turbulent Buoyant Jets: A Review of Experimental Data, Elsevier Science & Technology.
[33] Chen, Y. et al. (2020), “Experimental studies on external pressures during vented lean hydrogen deflagrations in a 27 m3 chamber”, Process Safety and Environmental Protection, Vol. 139, pp. 334–340.
[31] Chen, Y. et al. (2020), “Effects of hydrogen concentration, non-homogenous mixtures and obstacles on vented deflagrations of hydrogen-air mixtures in a 27 m3 chamber”, International Journal of Hydrogen Energy, Vol. 45/11, pp. 7199-7209, https://doi.org/10.1016/J.IJHYDENE.2019.11.082.
[29] Daubech, J. et al. (2015), “Un-ignited and ignited high pressure hydrogen releases: Concentration – turbulence mapping and overpressure effects,”, Journal of Loss Prevention in the Process Industries, Vol. 36, pp. 439-446.
[14] Ehrhart, B. et al. (2021), HyRAM+ (Hydrogen Plus Other Alternative Fuels Risk Assessment Models), Version 4.0. Sandia N.
[51] Giannissi, S. et al. (2015), “CFD benchmark on hydrogen release and dispersion in a ventilated enclosure: Passive ventilation and the role of an external wind”, International Journal of Hydrogen Energy, Vol. 40, pp. 6465-6477.
[52] Giannissi, S., I. Tolia and A. Venetsanos (2016), “Mitigation of buoyant gas releases in single-vented enclosure exposed to wind: Removing the disrupting wind effect”, International Journal of Hydrogen Energy, Vol. 41/6, pp. 4060-4071.
[6] Giannissi, S. et al. (2021), “On the CFD modelling of hydrogen dispersion at low-Reynolds number release in closed facility”, International Journal of Hydrogen Energy, Vol. 46/57, pp. 4060-4071.
[39] Groethe, M. et al. (2007), “Large-scale hydrogen deflagrations and detonations,”, International Journal of Hydrogen Energy, Vol. 32/13, pp. 2125–2133.
[15] Groth, K. and E. and Hecht (2017), “HyRAM: A methodology and toolkit for quantitative risk assessment of hydrogen systems”, International Journal of Hydrogen Energy, Vol. 42/11, pp. 7485–7493.
[12] Grune, J. et al. (2021), Hydrogen jet structure in presence of forced co-, counter- and cross-flow ventilation, Proceedings of 9th International Conference on Hydrogen Safety (ICHS 2021), 21-24 September 2021, ID41.
[3] Gupta, S. et al. (2009), “Hydrogen Related Risks within a Private Garage: Concentration Measurements in a Realistic Full Scale Experimental Facility”, International Journal of Hydrogen Energy, Vol. 34/14, pp. 5902–11.
[35] Hawthorne, W., D. Weddell and H. and Hottel (1949), Mixing and combustion in turbulent gas jets, Third International Symposium on Combustion, Flame and Explosion Phenomena.
[4] He, J. et al. (2016), “Assessment of similarity relations using helium for prediction of hydrogen dispersion and safety in an enclosure”, International Journal of Hydrogen Energy, Vol. 41, pp. 15388–15398.
[10] Hooker, P., J. Hoyes and J. and Hall (2014), Accumulation of Hydrogen Released into an Enclosure Fitted with Passive Vents - Experimental Results and Simple Models, Institution of Chemical Engineers Symposium Series.
[34] Hottel, H. and W. and Hawthorne (1949), “Diffusion in laminar flame jets”, Proceedings of the Combustion Institute, Vol. 3, pp. 254-266.
[25] Houf, G. et al. (2012), “Releases from hydrogen fuel-cell vehicles in tunnels”, International Journal of Hydrogen Energy, Vol. 37, pp. 715-719.
[50] HSE (2017), Fixed flammable gas detector systems on offshore installations: optimisation and assessment of effectiveness., RR1123.
[49] HyResponder (2021), Lecture 3 Hydrogen storage LEVEL IV Specialist Officer..
[11] IFV (2020), Safety aspects relating to the use of hydrogen in confined spaces.
[36] Kalghatgi, G. (1984), “Lift-off heights and visible lengths of vertical turbulent jet diffusion flames in still air”, Combustion Science and Technology, Vol. 41, pp. 17-29.
[40] Kuznetsov, M. et al. (2015), “Hydrogen combustion in a flat semi-confined layer with respect to the Fukushima Daiichi accident”, Nuclear Engineering and Design, Vol. 286, pp. 36–48.
[17] Lach, A. and A. Gaathaug (2021), “Effect of Mechanical Ventilation on Accidental Hydrogen Releases—Large-Scale Experiments”, Energies, Vol. 14, p. 3008.
[24] LaFleur, C. et al. (2017), Hydrogen fuel cell electric vehicle tunnel safety study.
[19] Lins, P. and A. de Almeida (2012), “Multidimensional risk analysis of hydrogen pipelines”, International Journal of Hydrogen Energy, Vol. 37/18, pp. 13545-13554, https://doi.org/10.1016/j.ijhydene.2012.06.078.
[37] Li, Y. (2018), Fire and explosion hazards of alternative fuel vehicles in tunnels..
[38] Li, Y. et al. (2021), “Numerical analysis of hydrogen release, dispersion and combustion in a tunnel with fuel cell vehicles using all-speed CFD code GASFLOW-MPI”, International Journal of Hydrogen Energy, Vol. 46, pp. 12474-12486.
[41] Makarov, D. et al. (2021), “Hydrogen tank rupture in fire in the open atmosphere: Hazard distance defined by fireball”, Hydrogen, Vol. 2/1, pp. 134-146.
[2] Messaoudani, Z. et al. (2016), “Hazards, safety and knowledge gaps on hydrogen transmission via natural gas grid: A critical review”, International Journal of Hydrogen Energy, Vol. 41/39, pp. 17511-17525, https://doi.org/10.1016/J.IJHYDENE.2016.07.171.
[26] Molkov, V. (2012), Fundamentals of Hydrogen Safety Engineering I, Bookboon.com Ltd.
[48] Molkov, V., D. Makarov and S. Kashkarov (2019), Composite vessel for hydrogen storage.
[20] Moosemiller, M. (2011), “Development of algorithms for predicting ignition probabilities and explosion frequencies”, Journal of Loss Prevention in the Process Industries, Vol. 24, pp. 259–265.
[5] Prabhakar, A. et al. (2017), “Numerical modelling of isothermal release and distribution of helium and hydrogen gases inside the AIHMS cylindrical enclosure”, International Journal of Hydrogen Energy, Vol. 42, pp. 15435–15447.
[18] Rigas, F. and A. P. (2013), Myths and facts about hydrogen hazards, 13th international symposium on loss prevention and safety promotion in the process industries.
[23] Rodsaetre, L. and K. Holmefjord (2007), An ignition probability model for hydrogen risk analysis, DNV, HySafe Deliverable No. 71.
[32] Schiavetti, M. and M. Carcassi (2021), “Experimental tests of inhomogeneous hydrogen deflagrations in the presence of obstacles”, International Journal of Hydrogen Energy, Vol. 46/23, pp. 12455-12463, https://doi.org/10.1016/J.IJHYDENE.2020.09.100.
[47] Shen, C. et al. (2018), “Consequence assessment of high-pressure hydrogen storage tank rupture during fire test”, Journal of Loss Prevention in the Process Industries, Vol. 55, pp. 223-231, https://doi.org/10.1016/J.JLP.2018.06.016.
[28] Skjold, T. et al. (2019), “Blind-prediction: Estimating the consequences of vented hydrogen deflagrations for inhomogeneous mixtures in 20-foot ISO containers”, Journal of Loss Prevention in the Process Industries, Vol. 61, pp. 220-236, https://doi.org/10.1016/J.JLP.2019.06.013.
[27] Skjold, T. et al. (2019), “Blind-prediction: Estimating the consequences of vented hydrogen deflagrations for homogeneous mixtures in 20-foot ISO containers”, International Journal of Hydrogen Energy, Vol. 44/17, pp. 8997-9008, https://doi.org/10.1016/J.IJHYDENE.2018.06.191.
[9] Skjold, T. et al. (2019), “Vented hydrogen deflagrations in containers: Effect of congestion for homogeneous and inhomogeneous mixtures”, International Journal of Hydrogen Energy, Vol. 44/17, pp. 8819-8832, https://doi.org/10.1016/J.IJHYDENE.2018.10.010.
[22] Tchouvelev, A. et al. (2006), Quantitative Risk Comparison of Hydrogen and CNG Refuelling Option, Final Technical Report to Natural Resources Canada for the Codes and Standards Workshop of the CTFCA.
[44] Weyandt, N. (2006), Vehicle Bonfire to Induce Catastrophic Failure of a 5000-Psig Hydrogen Cylinder Installed on a Typical SUV.
[42] Weyandt, N. (2005), Analysis of Induced Catastrophic Failure of A 5000 psig Type IV Hydrogen Cylinder.
[46] Yosuke, T. et al. (2006), “Fire Exposure Burst Test of 70MPa Automobile High-pressure Hydrogen Cylinders”, 自動車技術会学術講演会前刷集 115-06, pp. 7-10, https://jglobal.jst.go.jp/en/detail?JGLOBAL_ID=200902297707298027 (accessed on 10 May 2023).
[45] Zalosh, R. (23–27 April 2007), Blast waves and fireballs generated by hydrogen fuel tank rupture during fire exposure, University of Edinburgh.
[43] Zalosh, R. and N. Weyandt (2005), “Hydrogen Fuel Tank Fire Exposure Burst Test.”, SAE Trans, Vol. 114, pp. 2338–2343.
[21] Zhu, C., J. Jiang and X. Yuan (2012), “Study on ignition probability of flammable materials after leakage accidents”, Procedia Engineering, Vol. 45, pp. 435-441, https://doi.org/10.1016/J.PROENG.2012.08.182.
Notes
← 1. Riv=g*(ρα-ρ0)/ρ0*V1/3/U02.
where V is the volume of the facility, U the velocity, ρ the density, index α is for air and 0 for release conditions.
← 2. e-Laboratory is a virtual laboratory with tools for hydrogen behaviour and fuel cells (HFC) from a physical, an economic or a safety perspective. Free registration is required to get access.
← 3. Available at: https://energy.sandia.gov/programs/sustainable-transportation/hydrogen/hydrogen-safety-codes-and-standards/hyram/.
← 4. Hydrogen Plus Other Alternative Fuels Risk Assessment Models (HyRAM+) https://energy.sandia.gov/programs/sustainable-transportation/hydrogen/hydrogen-safety-codes-and-standards/hyram/.
← 5. Type III tanks: metallic liners fully wrapped with fibre resin composite.
← 6. Type IV tanks: polymeric liner fully wrapped with fibre resin composite (max. pressure 70 Mpa).